Prime Factorization

Prime Number:

A number which is divisible by 1 and by itself is called prime number.

Prime Factorization:

If a given number say 24 is divided by 2 we start getting the factors has 24= 2$\times$2 $\times$2 $\times$ 3. Here 2 and 3 are prime factors. So 24 = 2³ $\times$3.

Now let us learn to solve prime factorization with these below examples:

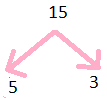
Prime Factors of 15

15 = 5$\times$3.
Step 1: Divide 15 by 3
Step 2: we get 5
so prime factors are illustrated below

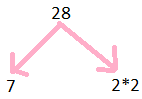
$Prime Factorization of 15$

Prime Factors of 28

28 = 2$\times$2$\times$7.
Step 1: Divide 28 by 2
Step 2: we get 14
Step 3: further divide by 2
Step 4: we get 7
so prime factors are illustrated below

$Prime Factorization of 28$

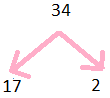
Prime Factors of 34

34 = 2$\times$ 17
Step 1: Divide 34 by 2
Step 2: we get 17
so prime factors are illustrated below

$Prime Factorization of 34$

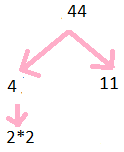
Prime Factorisation of 44

44 = 2$\times$2$\times$11
Step 1: Divide 44 by 2
Step 2: we get 22
Step 3: further divide by 2
Step 4: we get 11
so prime factors are illustrated below

$Prime Factorization of 44$

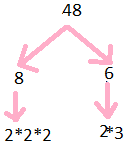
Prime Factorisation of 48

48 = 2$\times$2$\times$2$\times$2$\times$3
44 = 2$\times$2$\times$11
Step 1: Divide 48 by 2
Step 2: we get 24
Step 3: further divide by 2
Step 4: we get 12
Step 5: further divide by 2
Step 6: we get 6
Step 7: further divide by 2
Step 8: we get 3
so prime factors are illustrated below

$Prime Factorization of 48$

Prime Factorisation of 56

56= 2$\times$2$\times$2$\times$7
Step 1: Divide 56 by 2
Step 2: we get 28
Step 3: further divide by2
Step 4: we get 14
Step 5: further divide by 2
Step 6: we get 7
so prime factors are illustrated below

$Prime Factorization of 56$

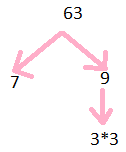
Prime Factorisation of 63

63= 3$\times$3$\times$9
Step 1: Divide 63 by 3
Step 2: we get 21
Step 3: further divide by 3
Step 4: we get 7
so prime factors are illustrated below

$Prime Factorization of 63$

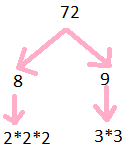
Prime Factorisation of 72

72= 2$\times$2$\times$2$\times$3$\times$3
Step 1: Divide 72 by 2
Step 2: we get 36
Step 3: further divide by 2
Step 4: we get 18
Step 5: further divide by 2
Step 6: we get 9
Step 7: divide it by 3
Step 8: we get 3
so prime factors are illustrated below

$Prime Factorization of 72$

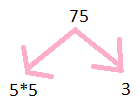
Prime factorisation of 75

75= 5$\times$5$\times$3
Step 1: Divide 75 by 5
Step 2: we get 15
Step 3: further divide by 5
Step 4: we get 3
Step 5: further divide by 3
Step 6: we get 1
so prime factors are illustrated below

$Prime Factorization of 75$

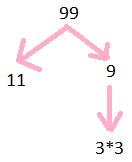
Prime Factorisation of 99

99 = 3$\times$3$\times$ 11
Step 1: Divide 99 by 3
Step 2: we get 33
Step 3: further divide by3
Step 4: we get 11
Step 5: further divide by 11
Step 6: we get 1
so prime factors are illustrated below

$Prime Factorization of 99$

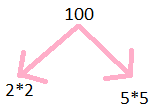
Prime factorisation of 100

100 = 2$\times$2$\times$5$\times$5
Step 1: Divide 100 by 5
Step 2: we get 20
Step 3: further divide by 5
Step 4: we get 4
Step 5: further divide by 2
Step 6: we get 2
Step 7: further divide by 2
Step 8: we get 1.
so prime factors are illustrated below

$Prime Factorization of 100$

Next Chapters

Number Theory	Linear Equation	Set Theory	Math Fractions
Math Functions	Pyramid	Calculus	Cone
Cylinder	Chain Rule	Limits and Continuity	Prime Factorization
Square Roots and Cube Roots	Parabola	Distance Formula	Definite Integrals
Interest	Simple Interest	Compound Interest	Area of Irregular Figures