Function

Any relation is said to be a function if there exists a coordination of each element from one set A to another set B

What is a function?

A relation is said to be a function for every element in set A their exits at least one element in B which is being mapped from A to B.

Example

$Venn Diagram$

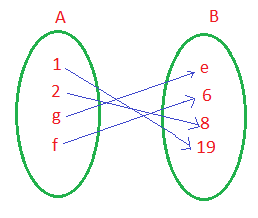
In the above example 1 is mapped to 19
2 is mapped to 6
g is mapped to 8
f is mapped to e

All the elements from set A is mapped to set B, so no element is left out. Hence the above example is a function.

Examples: To show the relation is not a function.

$Function Example$

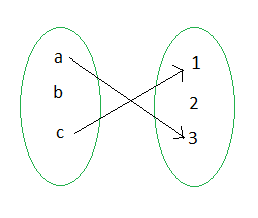
In the above example the element a is mapped to 3 and element c is mapped to 1 and b is not mapped at all hence it is necessary to map all the elements of set A to set B but it's not happening in the above example, hence the above one is not a function.

One-one function: All the elements from set A should have at least one element mapped to B is called one-one function or injective function.

Onto function: Every element in set B should have a pre image in B then we say that it is a onto function or subjective function.

Linear function

Linear functions are those which have the variable x and the degree of the variable is 1.

Example : f(x) = mx + c. Here x is having the exponent 1

Composition of a function

Composition of function refers to the combining of functions in such a way that the output from one function becomes the input for the next function.

The notation used for composition is:

f0g(x) = f{g(x).

It is read as fog.

Piece-wise functions: A piece-wise function is that function which is defined in different intervals. Example

$Piece Wise Functions$

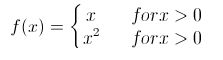
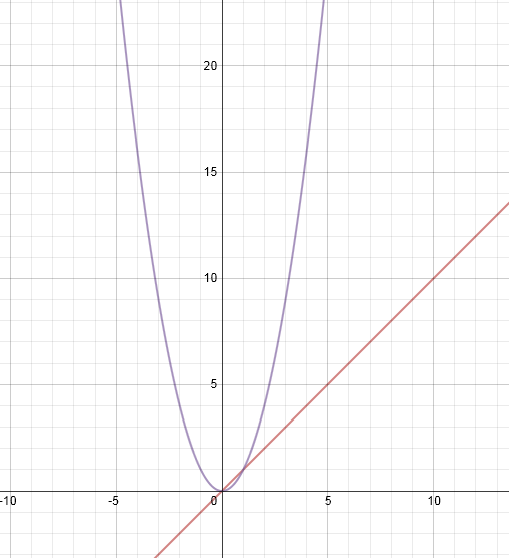
We will draw the graph for the above function for each interval separately and you will see that each function is discontinuous in its own way that is you can observe that y= x goes as a straight line from the origin and y= x^2 goes in +ve y -direction.

The graph of the above function is shown below:

$Piece Wise Function Graph$

Next Chapters

Number Theory	Linear Equation	Set Theory	Math Fractions
Math Functions	Pyramid	Calculus	Cone
Cylinder	Chain Rule	Limits and Continuity	Prime Factorization
Square Roots and Cube Roots	Parabola	Distance Formula	Definite Integrals
Interest	Simple Interest	Compound Interest	Area of Irregular Figures